
Paper: Approximate analytical solution for the pile-up (lip) profile in normal, quasi-static, elastoplastic, spherical and conical indentation of ductile materials.
Author: George K. Nikas
Published in: International Journal of Solids and Structures, 2022, 234-235C, 111240
Abstract
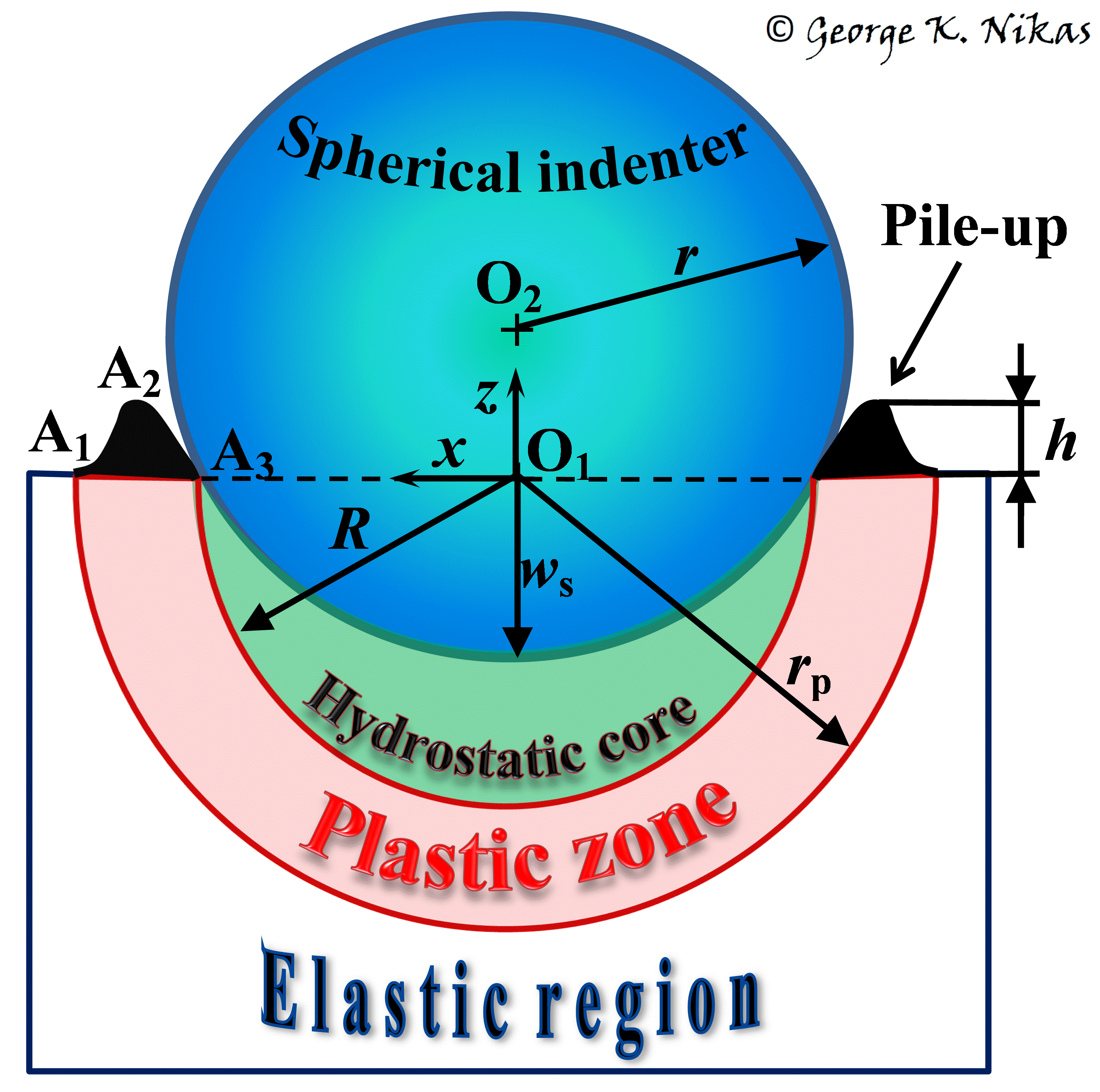
An approximate analytical formulation for the quasi-static, normal, elastoplastic indentation of ductile materials is presented. Explicit equations are derived, which describe the geometrical profile of material piling up above the original surface in cases of spherical and conical indentation. Cases of rigid, elastic, and plastic indenters are modelled. Surface bulging in such cases is known to be related to stress concentration and increased risk of damage in the form of crack initiation, spalling, and generation of sizeable wear debris when the dented surface is loaded against another surface. The developed equations offer a simple and robust method to include such critical phenomena in damage models. A major part of the study is devoted to experimentally validating the developed equations in spherical and conical indentation. The related comparisons show satisfactory to excellent agreement with experimental results, even in cases of micro-indentation. The algorithm of the method is also provided for easy implementation of the method by the reader.
Highlights